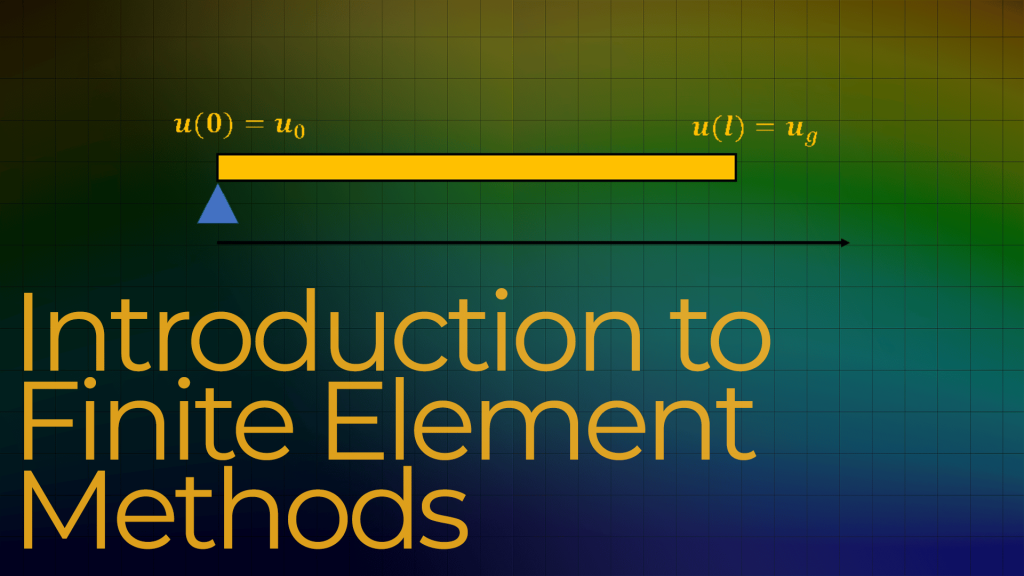
We begin this course with a discussion about boundary conditions. We discuss a pure Dirichlet problem using an example of a linear elastic bar. We then move on to developing higher-order polynomial basis functions for Lagrange polynomials and discuss some properties of Lagrange polynomials. We then derive the matrix-vector equations using quadratic basis functions. Finally, we discuss numerical integration and Gauss quadrature rules which help in solving the matrix-vector equations numerically.
This course was developed by Prof. Krishna Garikipati and Dr. Gregory Teichert, at the University of Michigan in partnership with Ansys.
STRUCTURES
Learn Physics
